Applying cubic spline to estimate definite integrals
Main Article Content
Abstract
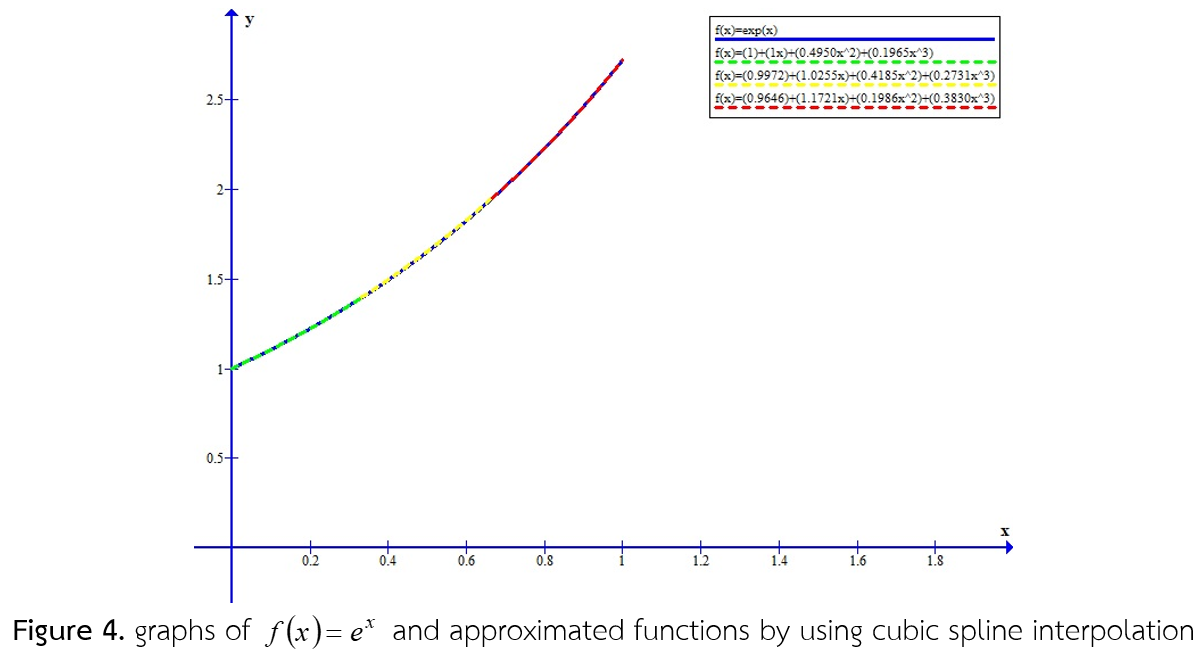
Evaluating definite integral is of mathematician interests, especially applying with non integrable functions. There are various ways to do so and one of them is evaluating a non analytic integrable function by using an analytic integrable function. If an estimated function is fairly good, we can replace the evaluating definite integral by the actual definite integral. This work aims to evaluating estimated functions by cubic spline method, its applications and definite integrals of the estimated functions. We also present evaluating functions by estimating them in intervals with cubic spline in both analytic integrable and non analytic integrable functions to focus clearly on the applications of using cubic spline for definite integrals.
Article Details
The Journal of Science and Science Education (JSSE) retain the right of all articles published in JSSE. The coresponding author or the authorized person on behalf of the authors must send the complete Copyright Transfer Form to JSSE before any article get published in JSSE.
Copyright Transfer Form
The JSSE request the coresponding author or the authorized person on behalf of the authors upload the manuscript under the together with the Copyright Transfer Form under the supplementary data. The guidline for uploading both manuscript and Copyright Transfer Form is shown below:
1. Upload the manuscript in the sub-menu, Article Component > Article Text.
2. Upload the the Copyright Transfer Form in the sub-menu, Article Component > Other.
Download Copyright Transfer Form
References
Bittinger, M. L., Ellenbogen, D. J. and Surgent, S. J. (2015). Calculus and its applications (10th ed.). Boston, MA: Pearson Education.
Burden, R. L. and Faires, J. D. (2001). Numerical Analysis (7th ed.). Stamford, CT: Thomson Learning.
Burt, P. J. and Adelson, E. H. (1983). A multiresolution spline with application to image mosaics. ACM transactions on Graphics, 2(4), 217-236.
Eldén, L. and Wittmeyer-Koch, L. (1990). Numerical Analysis: An Introduction. Boston, MA: Academic Press.
Giordano, F. R., Fox, W. P. and Horton, S. B. (2013). A First Course in Mathematical Modeling (5th ed.). Ontario: Nelson Education.
Heinbockel, J. H. (2012). Introduction to Calculus. Old Dominion University. Retrieved 6 Nov 2019, from http://www.math.odu.edu/~jhh/counter10.html
Kastanek, F. J. and Nielsen, D. R. (2001). Description of soil water characteristics using cubic spline interpolation. Soil Science Society of America Journal, 65(2), 279-283.
Kawamura, H., Sasaki, T. and Otsuki, T. (1992). Spline interpolation method. U.S. Patent No. 5,140,236. Washington, DC: U.S. Patent and Trademark Office.
McKinley, S. and Levine, M. (1998). Cubic spline interpolation. College of the Redwoods, 45(1), 1049-1060.
Scardapane, S., Scarpiniti, M., Comminiello, D. and Uncini, A. (2017, June). Learning activation functions from data using cubic spline interpolation. In Italian Workshop on Neural Networks (pp. 73-83). Retrieved 6 Nov 2019, from https://arxiv.org/pdf/1605.05509.pdf
Steven, C. C. (2007). Applied Numerical Methods with Matlab for Engineers and Scientists. New York: McGraw-Hill Higher Education
Wang, Y., Shen, D. and Teoh, E. K. (2000). Lane detection using spline model. Pattern Recognition Letters, 21(8), 677-689.