Mathematical modeling of stock market states using the system of difference equation
Main Article Content
Abstract
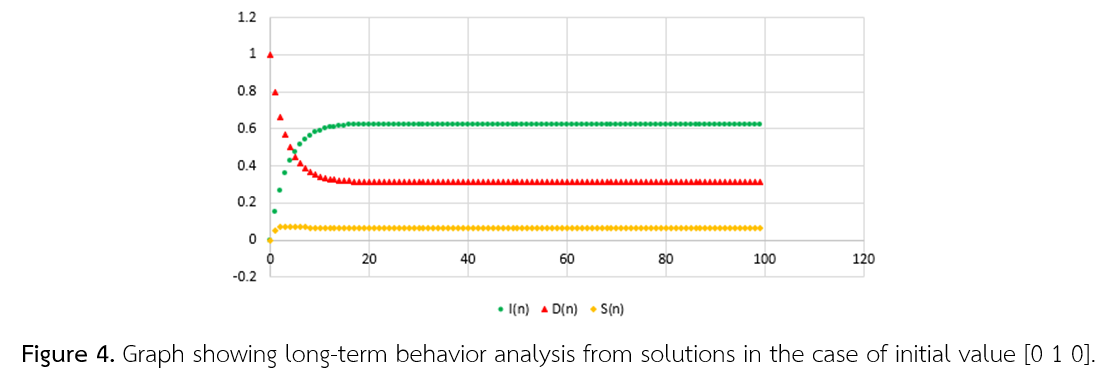
The objective of this research is to present the mathematical model of stock market states using the system of a difference equation. Beginning with the study of the analysis model in the Markov chain and converting it into a system of difference equations, which can be considered analytical solution different from finding the solution in the form of a Markov chain that requires a numerical method to find the numerical solution. In the process of determining the solution of the system of a difference equation the Wolfram Alpha program is used to help for finding the characteristic equation, eigenvalues, and eigenvectors and the use of Microsoft Excel to find the numerical solution and show the solution graph. A mathematical model about the stock market states is considered bull markets, bear markets and stagnant markets. Analysis of long-term behavior in the case of stocks studied in this the initial state of 3 cases are considered, which are [1 0 0], [0 1 0] , and [0 0 1]. The results of the study show that the long-term behavior of stock states initial with all 3 cases yields results. Likewise, there is a probability of a bull market status of 0.6250, a bear market of 0.3125 , and a stagnant market of 0.0625, suggesting that over time the stock studied has a probability of an uptrend, which will be useful mathematical information to be considered together with other trading data.
Article Details
The Journal of Science and Science Education (JSSE) retain the right of all articles published in JSSE. The coresponding author or the authorized person on behalf of the authors must send the complete Copyright Transfer Form to JSSE before any article get published in JSSE.
Copyright Transfer Form
The JSSE request the coresponding author or the authorized person on behalf of the authors upload the manuscript under the together with the Copyright Transfer Form under the supplementary data. The guidline for uploading both manuscript and Copyright Transfer Form is shown below:
1. Upload the manuscript in the sub-menu, Article Component > Article Text.
2. Upload the the Copyright Transfer Form in the sub-menu, Article Component > Other.
Download Copyright Transfer Form
References
Albright, B. and Fox, W. P. (2019). Mathematical modeling with Excel. Boca Raton, FL: CRC Press.
Andrieu, C., Doucet, A. and Holenstein, R. (2010). Particle markov chain monte carlo methods. Journal of the Royal Statistical Society: Series B (Statistical Methodology), 72(3), 269-342.
Bidabad, B. and Bidabad, B. (2019). Complex probability and Markov stochastic process. Indian Journal of Finance and Banking, 3(1), 13-22.
Brooks, S., Gelman, A., Jones, G. and Meng, X. L. (Eds.). (2011). Handbook of Markov chain Monte Carlo. Boca Raton, FL: CRC press.
Fox, W. P. (2011). Mathematical modeling with Maple. Nelson Education, Belmont, CA: Brooks/Cole Publishing.
Giordano, F. R., Fox, W. P. and Horton, S. B. (5ed, 2013). A first course in mathematical modeling (5th ed.), Nelson Education , Belmont, CA: Brooks/Cole Publishing.
Myers, D., Wallin, L., Wikström, P. (2017) An introduction to Markov chains and their applications within finance. Sweden: Mathematical Sciences - Chalmers University of Technology and University of Gothenburg, Retrieved 10 September 2020, from Chalmers tekniska högskola och Göteborgs Universitet: http://www.math.chalmers.se/Stat/Grundutb/CTH/mve220/1617/redingprojects16-17/IntroMarkovChainsandApplications.pdf, 21. 03. 2019, str. 1-8
Svoboda, M. and Lukas, L. (2012). Application of Markov chain analysis to trend prediction of stock indices. In Proceedings of 30th International Conference Mathematical Methods in Economics (pp. 848-853). Karviná: Silesian University, School of Business Administration.
Vasanthi, S., Subha, M. V. and Nambi, S. T. (2011). An empirical study on stock index trend prediction using Markov chain analysis. Journal of Banking Financial Services and Insurance Research, 1(1), 72-91.
Yu, K. and Sato, T. (2019). Modeling and analysis of error process in 5G wireless communication using two-state Markov chain. IEEE Access, 7, 26391-26401.
Zhang, D. and Zhang, X. (2009). Study on forecasting the stock market trend based on stochastic analysis method. International Journal of Business and Management, 4(6), 163-170.