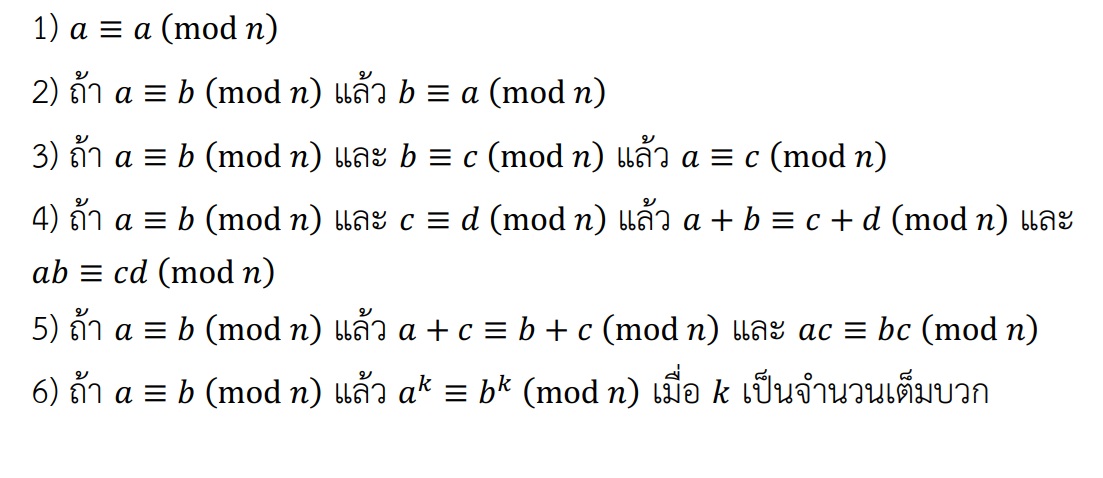ผลเฉลยของสมการไดโอแฟนไทน์ 〖11〗^x-3^y=z^2 และ 〖14〗^x-3^y=z^2
Main Article Content
บทคัดย่อ
ในงานวิจัยนี้ได้ศึกษาผลเฉลยทั้งหมดของสมการไดโอแฟนไทน์ และ
เมื่อ
และ
เป็นจำนวนเต็มที่ไม่เป็นลบ โดยใช้ความรู้พื้นฐานของสมภาคและทฤษฎีบทของมิไฮเลสคู ผลการวิจัย พบว่า สมการไดโอแฟนไทน์
มีผลเฉลยจำนวนเต็มที่ไม่เป็นลบ
เพียงผลเฉลยเดียว คือ
และสมการไดโอแฟนไทน์
มีผลเฉลยจำนวนเต็มที่ไม่เป็นลบ
เพียงสองผลเฉลย คือ
และ
Article Details
References
Burshtein, N. 2020. All the solutions of the Diophantine equations 〖13〗^x-5^y=z^2 ,〖19〗^x-5^y=z^2 in positive integers x,y,z. Annals of Pure and Applied Mathematics, 22(2): 93-96.
Burton, D.M. 2010. Elementary number theory. 7th ed., New York: McGraw-Hill.
Chuayjan, W., Thongnak, S. and Kaewong, T. 2023. On the exponential Diophantine equation 3^x-5^y=z^2. Annals of Pure and Applied Mathematics, 28(1): 25-28.
Gope, R.C. 2023. On the exponential Diophantine equation 〖27〗^x-〖11〗^y=z^2 . Journal of Physical Sciences, 28: 11-15.
Kaewong, T., Chuayjan, W. and Thongnak, S. 2023. On the exponential Diophantine equation 〖29〗^x-3^y=z^2. International Journal of Latest Technology in Engineering, Management & Applied Science, 12(11): 74-76.
Mihăilescu, P. 2004. Primary cyclotomic units and a proof of Catalan’s conjecture. Journal für die Reine und Angewandte Mathematik, 572: 167-195.
Rao, C.G. 2022. On the exponential Diophantine equation 〖23〗^x-〖19〗^y=z^2 . Journal of Physical Sciences, 27: 1-4.
Siraworakun, A. and Tadee, S. 2024. All solutions of the Diophantine equation 〖25〗^x-7^y=z^2. International Journal of Mathematics and Computer Science, 19(3): 631-633.
Tadee, S. 2023. A short note on two Diophantine equations 9^x-3^y=z^2 and 〖13〗^x-7^y=z^2. Journal of Mathematics and Informatics, 24: 23-25.
Thongnak, S., Chuayjan, W. and Kaewong, T. 2019. On the exponential Diophantine equation 2^x-3^y=z^2. Southeast-Asian Journal of Sciences, 7(1): 1-4.
Thongnak, S., Chuayjan, W. and Kaewong, T. 2021. The solution of the exponential Diophantine equation 7^x-5^y=z^2. Mathematical Journal by The Mathematical Association of Thailand under the Patronage of His Majesty the King, 66(703): 62-67.
Thongnak, S., Chuayjan, W. and Kaewong, T. 2022. On the Diophantine equation 7^x-2^y=z^2 where x,y and z are non-negative integers. Annals of Pure and Applied Mathematics, 25(2): 63-66.
Thongnak, S., Kaewong, T. and Chuayjan, W. 2024. On the exponential Diophantine equation 5^x-3^y=z^2. International Journal of Mathematics and Computer Science, 19(1): 99-102.