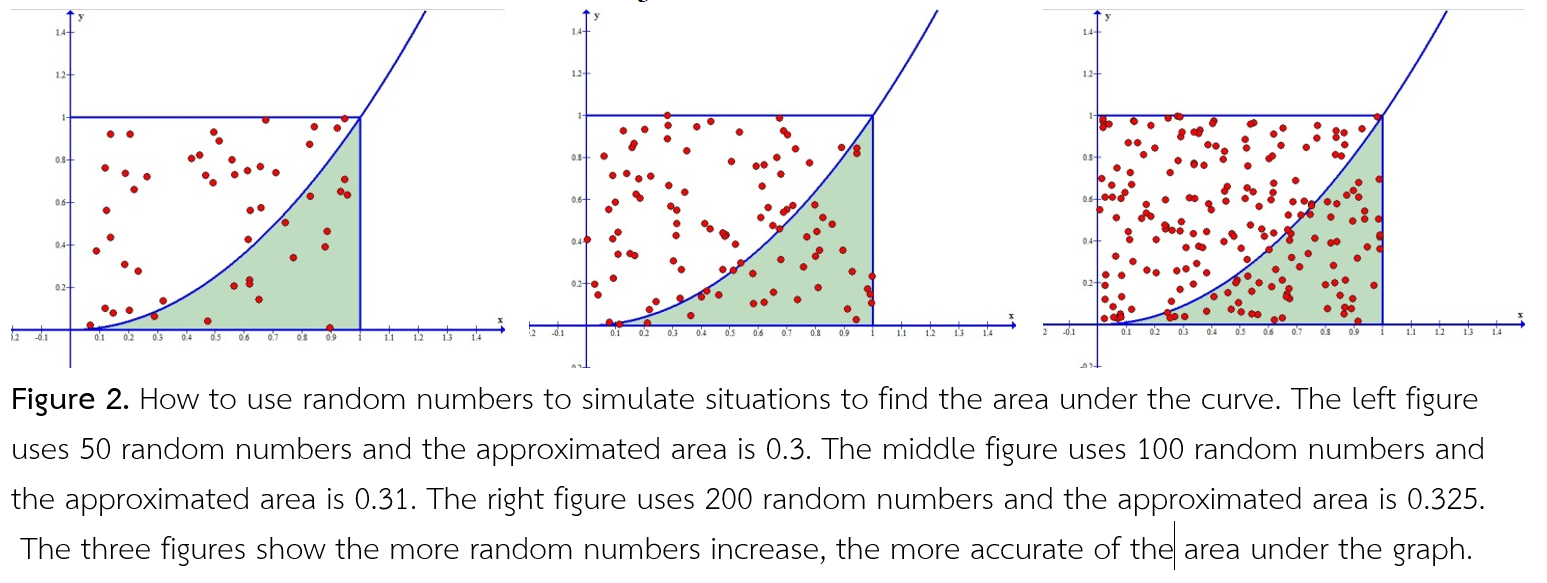
การใช้เลขสุ่มเพื่อจำลองสถานการณ์
Main Article Content
บทคัดย่อ
การประยุกต์คณิตศาสตร์มาใช้เพื่ออธิบายปรากฏการณ์ทั้งหลายที่เกิดขึ้น ซึ่งเป็นที่รู้จักกันในนามตัวแบบเชิงคณิตศาสตร์นั้น มีประโยชน์อย่างยิ่งต่อการนำไปประยุกต์ใช้ในทุกด้าน ถ้าหากตัวแบบเชิงคณิตศาสตร์ที่สร้างขึ้นมานั้นสามารถอธิบายปรากฏการณ์เหล่านั้นได้แม่นยำ การใช้เลขสุ่มเพื่อจำลองสถานการณ์ถือว่าเป็นการสร้างตัวแบบเชิงคณิตศาสตร์อีกแบบหนึ่งที่มีความสำคัญ และประยุกต์ใช้กันอย่างแพร่หลาย บทความนี้มีจุดประสงค์เพื่อนำเสนอการใช้เลขสุ่มเพื่อจำลองสถานการณ์ มีการอธิบายให้เห็นถึงการใช้เลขสุ่มเพื่อจำลองสถานการณ์เชิงกำหนดและสถานการณ์เชิงความน่าจะเป็นที่ง่ายต่อการเข้าใจ มีการสาธิตการประยุกต์การใช้เลขสุ่มเพื่อจำลองสถานการณ์ในชีวิตประจำวันจริงเกี่ยวกับคงคลังสินค้า ผลลัพธ์ที่ได้เป็นอีกหนึ่งแนวทางในการนำคณิตศาสตร์ไปประยุกต์ใช้จริงเพื่อช่วยให้การวางแผนจัดการเกี่ยวกับสถานการณ์ทั้งหลายให้มีประสิทธิภาพมากขึ้น โดยอาศัยข้อมูลที่มาจากตัวแบบเชิงคณิตศาสตร์ที่สร้างขึ้น
Article Details
วารสารวิทยาศาสตร์และวิทยาศาสตร์ศึกษา (JSSE) เป็นผู้ถือลิสิทธิ์บทความทุกบทความที่เผยแพร่ใน JSSE นี้ ทั้งนี้ ผู้เขียนจะต้องส่งแบบโอนลิขสิทธิ์บทความฉบับที่มีรายมือชื่อของผู้เขียนหลักหรือผู้ที่ได้รับมอบอำนาจแทนผู้เขียนทุกนให้กับ JSSE ก่อนที่บทความจะมีการเผยแพร่ผ่านเว็บไซต์ของวารสาร
แบบโอนลิขสิทธิ์บทความ (Copyright Transfer Form)
ทางวารสาร JSSE ได้กำหนดให้มีการกรอกแบบโอนลิขสิทธิ์บทความให้ครบถ้วนและส่งมายังกองบรรณาธิการในข้อมูลเสริม (supplementary data) พร้อมกับนิพนธ์ต้นฉบับ (manuscript) ที่ส่งมาขอรับการตีพิมพ์ ทั้งนี้ ผู้เขียนหลัก (corresponding authors) หรือผู้รับมอบอำนาจ (ในฐานะตัวแทนของผู้เขียนทุกคน) สามารถดำเนินการโอนลิขสิทธิ์บทความแทนผู้เขียนทั้งหมดได้ ซึ่งสามารถอัพโหลดไฟล์บทความต้นฉบับ (Manuscript) และไฟล์แบบโอนลิขสิทธิ์บทความ (Copyright Transfer Form) ในเมนู “Upload Submission” ดังนี้
1. อัพโหลดไฟล์บทความต้นฉบับ (Manuscript) ในเมนูย่อย Article Component > Article Text
2. อัพโหลดไฟล์แบบโอนลิขสิทธิ์บทความ (Copyright Transfer Form) ในเมนูย่อย Article Component > Other
ดาวน์โหลด ไฟล์แบบโอนลิขสิทธิ์บทความ (Copyright Transfer Form)
References
Al-Harkan, I. and Hariga, M. (2007). A simulation optimization solution to the inventory continuous review problem with lot size dependent lead time. Arabian Journal for Science and Engineering, 32(2), 327-338.
Badri, M. A. (1999). A simulation model for multi-product inventory control management. Simulation, 72(1), 20-32.
Davis, E. R., Eckhause, J. M., Peterson, D. K., Pouy, M. R., Sigalas-Markham, S. M. and Volovoi, V. (2013). Exploring how hierarchical modeling and simulation can improve organizational resourcing decisions. In Proceedings of the Winter Simulation Conference: Simulation: Making Decisions in a Complex World (pp. 2496-2507). Piscataway, NJ: IEEE Press.
Giordano, F. R., Fox, W. P. and Horton, S. B. (2013). A first course in mathematical modeling. Nelson Education.
Ižaríková, G. (2015). Process simulation and method of generating random numbers. International Scientific Journal about Simulation, 1(2), 1-4.
L'Ecuyer, P. (1990). Random numbers for simulation. Communications of the ACM, 33(10), 85-97.
Lee, E. and Farahmand, K. (2010). Simulation of a base stock inventory management system integrated with transportation strategies of a logistic network. In Proceedings of the Winter Simulation Conference (pp. 1934-1945). Piscataway, NJ: IEEE Press.
Merkuryev, Y. A., Petuhova, J. J., Van Landeghem, R. and Vansteenkiste, S. (2002). Simulation-based analysis of the bullwhip effect under different information sharing strategies. In Proceedings of European Simulation Symposium (Vol. 299). Piscataway, NJ: IEEE Press.
Metropolis, N. (1987). The beginning of the Monte Carlo method. Los Alamos Science, 15(584), 125-130.
Robinson, S. (1997). Simulation model verification and validation: increasing the users' confidence. In Proceedings of the Winter Simulation Conference (pp. 53-59). Washington, DC: IEEE Computer Society.